Answers to problems in "Electrode Potentials"

Question 8

ERRATUM: Kw = aH+ aOH- and not as printed in the book.

Both cells A and B are Harned Cells

Pt | H2(g) | HCl(aq) | AgCl(s) | Ag(s)
Potential determining Equilibria:
RHE: AgCl(s) + e- ⇌ Ag(s) + Cl-(aq)

LHE: H+(aq) + e- ⇌ ½H2(g)

Cell Reaction:

AgCl(s) + ½H2(g) ⇌ Ag(s) + H+(aq) + Cl-(aq)

Nernst Equation: Ecell = E0cell - {RT/F}ln{aH+ aCl-},

for PH2 = 1 bar which approximates to 1 atm., and where E0cell = E0Ag|AgCl (by definition of E0H+|H2 = 0V).

Consider Cell A

aH+ = Kw/ aOH-

Hence, Ecell = E0Ag|AgCl - {RT/F}ln{Kw aCl-/ aOH-}

Now, aX = fX[X], where fX is the activity coefficient of X (also given the lower case Greek symbol gamma) and, [Cl-] = [OH-] = c

Also, since c < 10-2M, the Debye-Hückel Limiting Law (DHL) applies.

Since lgfi = -Azi2I½, and I, zi2 and A are all the same for both Cl- and OH-, fCl- = fOH- and so,

Ecell = E0Ag|AgCl - {RT/F}lnKw (1)

From the data given, we notice that Ecell A is not a function of concentration (as equation (1) predicts), and therefore,

1.0493 = E0Ag|AgCl - {RT/F}lnKw (2)

We next need a value for E0Ag|AgCl which (if we are to find Kwmust therefore be obtained from cell B.

Now we consider Cell B:

Ecell = E0Ag|AgCl - {RT/F}ln{aH+ aCl-}

aH+ = f+c and aCl- = f-(2c)

Using the DHL, we see that f+ = f- = f , since zi2, A(= 0.509 in water at 298K) and I are the same for both H+ and Cl-, as they co-exist in a solution of ionic strength I.

I = ½(c + 2c) = 3c/2 mol dm-3

Therefore, E = E0Ag|AgCl - {RT/F}ln{f2 2c2}

E + {RT/F}ln{2c2} = E0Ag|AgCl - {4.606RT/F}lg(f)

E + {RT/F}ln{2c2} = E0Ag|AgCl - 4.606(0.509)RT(3/2)½ c½/ F

Hence, a plot of E + {RT/F}ln{2c2} (on the ordinate axis) against c½ (on the abscissa) will have an ordinate intercept equal to the Standard Electrode Potential for Ag|AgCl.

E/V c/mol dm-3 c½/ mol½ dm-3/2 E + {RT/F}ln{2c2}/V

0.578

0.001 0.0316 0.2411

04969

0.005 0.0707 0.2426

0.4624

0.010 0.1000 0.2437

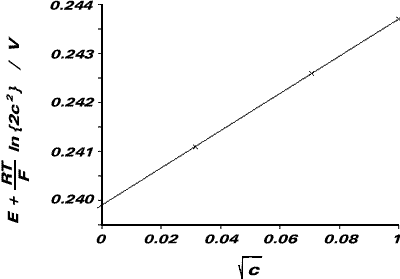
From the graph, ordinate intercept = E0Ag|AgCl = +0.2395V

Thus, from (2), Kw = 2.00 x 10-14 mol2 dm-6.

Of the course the true answer for Kw is only one half as big; students 'refining' their results beware!