Answers to problems in "Electrode Potentials"
Question 10
| Cd(amalgam, 4.6% Cd) | CdCl2(aq, cM) | AgCl(s) | Ag(s) |
Potential determining equilibria:
|
RHE: |
AgCl(s) + e- ⇌ Ag(s) + Cl-(aq) |
|
LHE: |
½Cd2+ + e- ⇌ ½Cd(amal) |
Cell Reaction:
AgCl(s) + ½Cd(amal) ⇌ Ag(s) + ½Cd2+(aq) + Cl-(aq)
The Nernst Equation for this cell reaction is: E = E0cell - {RT/F}ln {aCl- aCd2+½}
(where the activity of cadmium in the amalgam is taken to be unity).
aCl- = f-(2c) and aCd2+ = f+(c). Since c < 10-2M, we can use the DHL. The ionic strength of the solution, I = ½[ 4c +2c] = 3c. Since this is the same for both Cd2+ and Cl-, and since the ratio
zCd2+2/zCl-2
= 4, we can deduce that lg f+/lg f- = 4. ie.,
f+ = f-4. We can use this result
to determine E0cell. However, it is easier
and more convenient to use the mean ionic activity coefficient,
f±, which is defined on p.48.
Hence, aCl- = f±(2c)
= f±[Cl-] and aCd2+
= f±(c) = f±[Cd2+]
Therefore, E = E0cell - {RT/F}ln{f±3/2
(2)(c)3/2}
or,
| E + {RT/F}ln{2c3/2} = E0cell - {3 RT/ 2F}ln{f±} | (*) |
or, using DHL, E + {RT/F}ln{2c3/2} = E0cell - {6.909 RT A 31/2 / 2F} c1/2
We plot a graph of E + {RT/F}ln{2c3/2} against c1/2, and extrapolate to find the ordinate intercept (ie. a value for E0cell). The cell emf when aCd2+ = aCl- = 1 corresponds to Ecell.
| c / 10-3 mol dm-3 | E / V | c1/2 / mol1/2 dm-3/2 | E + {RT/F}ln{2c3/2} / V |
|
0.1087 |
0.9023 | 0.01041 | 0.56861 |
|
0.1269 |
0.8978 | 0.01126 | 0.57007 |
|
0.2144 |
0.8803 | 0.01464 | 0.57277 |
|
0.3659 |
0.8641 | 0.01913 | 0.57715 |
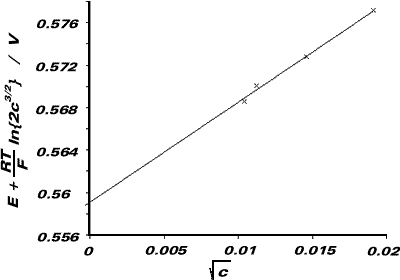
From graph, ordinate-intercept = E0cell = +0.559V
(a). E0cell = E0Ag|AgCl - E0Cd|Cd(amal)
Therefore, E0Cd|Cd(amal) = 0.2222
- 0.559 = -0.3369V
Now consider the cell:
| Cd(amal, 4.6% Cd) | CdCl2(aq, 0.5M) | Cd | E = -0.0534V |
For this cell, E0cell = E0Cd|Cd2+ - E0Cd2+|Cd(amal)
Cell Reaction is:
Cd2+(aq) + Cd(amal) Cd(s)
+ Cd2+(aq)
Thus, the Nernst Equation becomes: E = E0cell + {RT/ 2F} ln {aCd2+/ aCd2+}
E = E0cell
Hence, E0Cd|Cd2+ = -0.0534 + (-0.3369) = -0. 39V
Therefore, the S.E.P. for the Cd|Cd2+ couple is -0.39V
This result is consistent with the data supplied in Table 1.1 on p. 21.
(b). The Gibbs free energy of the formation of the
hydrated ion Cd2+(aq) refers to the process:
But this process corresponds to the inverse of the Cd|Cd2+ S.E.P.
Since DG0 = -nFE0,
and this process corresponds to a two electron transfer (n = 2),
DG0 = -(2)(96 490)(0.3903)
DG0 = -75.32 kJ mol-1
(c). Using the equation labelled with an asterix (*), we can deduce that the mean ionic activity coefficient (f±) of 0.2144 x 10-3 M CdCl2 is 0.701.


