QUESTION 12

(i) The half-cell reaction for the anthraquinone monosulphonate redox-couple is

1/2 A(aq) + e^- + H⁺ ⇌ 1/2 AH₂ (aq)

which implies the following electrochemical cell

Pt|H₂(g) (P=1 atm) | H⁺(aq) (a=1) ||A(aq)(a_A), H⁺(aq) (a_H⁺), AH₂ (aq) (a_AH2) |Pt

The corresponding Nernst equation is as follows noting that the hydrogen electrode above is standard

                                              E = E⁰_A/AH2 + RT/F ln[(a_A^1/2 a_H⁺)/( a_AH2^1/2)]

Since anthraquinone and anthrahydroquinone are neutral molecules and uncharged their activities can, to a good degree of accuracy, by approximated by their concentrations. The Nernst equation is simplified to

                                              E = E⁰_A/AH2 + RT/F ln[ ([A]^1/2 a_H⁺)/( [AH₂]^1/2) ]

 

(ii) The Nernst equation from (i) can be written as

E = E⁰_A/AH2 + RT/F ln[ ([A]^1/2 a_H⁺)/( [AH₂]^1/2) ] + RF/T ln(a_H⁺)

Substituting log₁₀N = lnN/ln(10) into the above gives

E = E⁰_A/AH2 + RT/F ln[ ([AH₂]^1/2)/( [A]^1/2) ] + ln(10)RF/T log₁₀(a_H⁺)

Noting that pH = -log₁₀(a_H⁺), the Nernst equation can be written in terms of pH

E = E⁰_A/AH2 + RT/F ln[ ([AH₂]^1/2)/( [A]^1/2) ] + ln(10)RF/T pH                  

Differentiating the above equation with respect to pH gives

                                     dE/d(pH) = -ln(10)RT/F

At 25°C, T = 298K.

                                     dE/d(pH) = -ln(10) x 298 x 8.31 / 96485.3 = -59mV per pH unit

 

(iii) The generalised redox process

B + ne^- + mH⁺ ⇌ BH_m

can be written as an one electron half-cell reaction

1/n B + e^- + m/n H⁺ ⇌ 1/n BH_m

The Nernst equation for the generalised redox process is therefore

E = E⁰_B/BHm+ RT/F ln[ (a_B^1/n a_H+^m/n)/( a_BHm^1/n) ]

E = E⁰_B/BHm+ RT/F ln[ (a_B^1/n)/( a_BHm^1/n) ] + RF/T ln(a_H+^m/n)

E = E⁰_B/BHm+ RT/F ln[ (a_B^1/n)/( a_BHm^1/n) ] + mRF/nT ln(a_H+)

E = E⁰_B/BHm+ RT/F ln[ (a_B^1/n)/( a_BHm^1/n) ] + mRF/nT log₁₀(a_H+)

Substituting in pH = -log₁₀(a_H⁺) gives

E = E⁰_B/BHm+ RT/F ln[ (a_B^1/n)/( a_BHm^1/n) ] + mRF/nT pH

Differentiating the above equation with respect to pH gives

dE/d(pH) = - mRT/nF ln(10)

 

(iv) Acid dissociation constant, K_A, for the dissociation reaction HA⇌ H⁺ + A^-  is given by

K_A = a_H⁺ a_A^- / a_HA

 

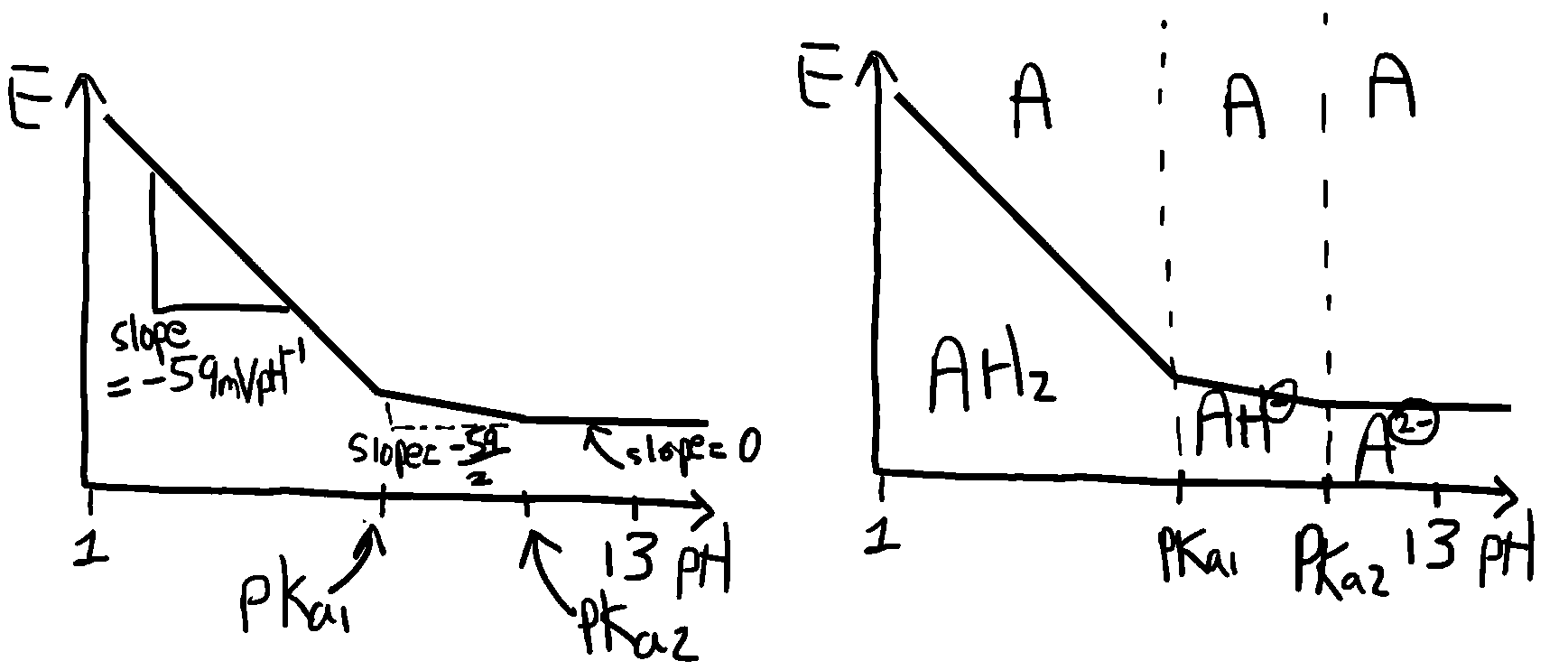
(v) For A/AH₂ system (e.g. anthraquinone/anthrahydroquinone), m = 2, n = 2

                               slope  = dE/d(pH) = 2RT/2nF ln(10) = -59mV per pH unit

                              pKa₁ (AH^-/AH₂) = -log(2x10^-8)=7.7; pKa₂ (A^2-/AH^-) = -log(1.2x10^-11)=10.9

Accordingly, the reduced species (anthrahydroquinone) exists predominantly as AH₂ for pH less than 7.7, as AH^- in the pH range 7.7 to 10.9 and as A^2- for pH > 10.9. Applying the results derived above leads to the following E – pH behaviour using the value n = 2 throughout but m = 0, 1 or 2 as appropriate.

 

(vi, vii)